Adding and Subtracting Fractions with Mixed Numbers: Representational Level

More Teaching Plans on this topic: Concrete, Abstract

PHASE 2: Practice Strategies

Receptive/Recognition Level

Purpose: to provide students multiple opportunities to practice matching equations to their solution represented on place value mats.

Learning Objective1: Add fractions with mixed numbers using a “fraction place value mat” and a fraction number line.

Self-Correcting Materials – Flash Cards

Materials:

Teacher –

• Sets of 5 x 7 note-cards with the following: Front – an equation written at the top with two or three examples of place value mats containing the numbers represented in appropriate columns and a solution. A hole is punched next to each choice. Back – a star or other cue is drawn next to the hole that represents the correct solution.

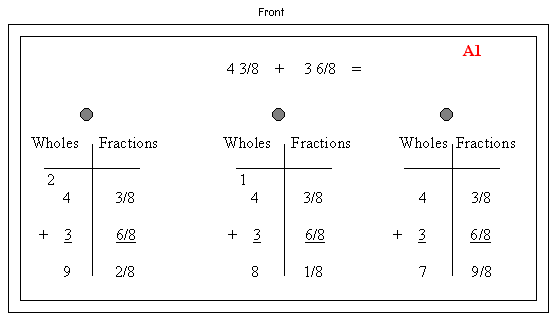
*To make the card development process easier, you can develop the equations and choices by writing them on sheets of copy paper to fit the size of the note-cards you are using. For example, the above equation and choices can be made so that if cut out, they can be glued to the front side of a 5 x 7 note-card. Multiple copies of examples and choices can be made to make multiple sets of cards. Code each set of cards by representing each set with a letter and each card with the letter of the set and a number that represents the particular example/problem. Answer keys can be developed based on the letter and number for each card. You can make multiple sets of the same problems or have several sets of note-cards that represent different problems.

• Answer key for each lettered set of cards.

Students -

• One set of note cards.
• Sheet of paper. (Students can number the paper as they respond to the note-cards. They also should put the letter of the set at the top of their response sheet.)
• Pencil

Description:

Activity:

Students work individually with sets of 5 x 7 note cards with appropriate prompts and choices on the front side and the answer provided on the backside. Students read the equation at the top of the card and then examine the three choices underneath the equation. Each choice is a fraction place value mat with the equation represented as well as the solution. Students choose the place value mat that represents the correct solution by placing the point of their pencil in the hole next to their choice. They turn the card over to see if the hole they chose has a “star” or some other cue indicating the correct solution. Students keep track of their responses by writing their answers on a numbered sheet of notebook paper. Students also write the “letter” of the set of cards they use found at the upper right hand corner of each card. The teacher monitors students as they practice by providing specific corrective feedback, positive reinforcement, and answering questions as needed. After the activity, students turn their response sheets in and the teacher evaluates them using the answer key for each set of note-cards to check for student understanding.

Self-correcting Materials Steps:

1) Introduce self-correcting material.

2) Distribute materials.

3) Provide directions for self-correcting material, what you will do, what students will do, and reinforce any behavioral expectations for the activity.

4) Provide time for students to ask questions.

5) Model responding/performing skill within context of the self-correcting material.

6) Model how students can keep check their responses.

7) Have students practice one time so they can apply what you have modeled. Provide specific feedback/answer any additional questions as needed.

8) Monitor students as they work.

9) Provide ample amounts of positive reinforcement as students play.

10) Provide specific corrective feedback/ re-model skill as needed.

11) Encourage students to review their individual learning sheets, write the total number of “correct” responses under the “C” (Correct) column and do the same for the “H” (Help) column.

12) Review individual student performance record sheets.

Expressive Level

Purpose: to provide students multiple opportunities to practice adding fractions with mixed numbers.

Learning Objective1: Add fractions with mixed numbers using a “fraction place value mat” and a fraction number line.

Instructional Game – Fraction Dice Game

Materials:

Teacher –

• Pairs of die: Each pair includes one die that has whole numbers on each side, while the other die has fractions with like denominators on each side. Blank labels can be used to make numbers and fractions. Cut out a piece of label the fits the side of a die and write the appropriate whole number or fraction on it. Glue the numbers and fractions to the sides of the dice. *See “Alternative Ideas for Prompts” under “Description” for additional ideas.
• Game board (optional)
• Sets of Chance cards with different consequences written on them (e.g. add 3pts to your score; move ahead 2 spaces; subtract 2 pts from your score, move back 3 spaces.)

Students -

• Two pair of dice; two spinners; two sets of cards depicting whole numbers and fractions with like denominators.
• One game board per small group (optional)
• One set of Chance Cards per small group
• Each student has a copy of a DRAW Strategy Cue Sheet (includes steps of the DRAW Strategy.)
• Each student two sheets of notebook paper with one labeled “play” and one labeled “check.”
• Pencil

Description:

Activity:

Students can play in pairs or small groups. Each small group has two pair of dice. One die has whole numbers on each side (e.g. 1, 2, 3, 4, 5, 6) and one die has fractions with like denominators (e.g. ¼, 2/4, ¾, 4/4). Each player roles each pair of dice one at a time. With each role, the player writes the mixed number represented by the pair of dice in the form of an addition equation on their “play” sheet. The player adds the two mixed numbers using a DRAW Strategy Cue Sheet as needed and writes the answer. The other players in the small group check the player’s response by solving the equation on their “check” sheet. For correct responses, each player can either receive a set number of points or move a game piece on a game board. The winner is the student who reaches a designated total of points or who reaches the finish space on the game board. Chance” cards can also be included to increase motivation. Students select a “chance” card after they respond. Each “chance” card either tells the player to add bonus points to their score/move additional spaces, or it tells them to subtract points from their score/move back a specified number of spaces. The teacher monitors student academic and social behavior as they practice by providing positive reinforcement, providing specific corrective feedback, and answering questions as needed. Students turn in both their “play” sheets and their “check” sheets at the end of the activity. The teacher reviews individual sheets to evaluate student understanding.

Alternative Ideas for Prompts:

Two spinners can also be used, where one spinner has whole numbers listed and the other has fractions with common denominators listed (Spinners can be made so they have multiple sets of fractions with like denominators. A circular spinner can be divided into “bands” of fourths, sixths, and eighths where the outside “band” has sections with “fourths,” the middle band has sections with “sixths,” and the inside “band” has sections with “eighths.” Students can be assigned particular fractions with like denominators.) Another alternative is sets of cards that have written whole numbers and fractions. Students pull one card from the whole number pile and one card from the fraction pile for each mixed number.

Instructional Game Steps:

1) Introduce game.

2) Distribute materials.

3) Provide directions for game, what you will do, what students will do, and reinforce any behavioral expectations for the game.

4) Provide time for students to ask questions.

5) Model how to respond to the prompts.

6) Provide time for students to ask questions about how to respond.

7) Model how students can keep track of their responses, how to check other student’s responses, and how to give and receive corrective feedback.

8) Play one practice round so students can apply what you have modeled. Provide specific feedback/answer any additional questions as needed.

9) Monitor students as they practice by circulating the room, providing ample amounts of positive reinforcement as students play, providing specific corrective feedback/ re-modeling skill as needed.

10) Play game.

11) Encourage students to review their individual response sheets, write the total number of “correct” responses under the “C” (Correct) column and do the same for the “H” (Help) column.

12) Review individual student response sheets to determine level of understanding/proficiency and to determine whether additional modeling from you is needed.