|
Metacognitive Strategies
Introduction
This site provides a detailed description of metacognitive strategies. This site also explains why metacognitive strategies are helpful for students who have learning problems, it provides tips on teaching metacognitive strategies, it includes a step-by-step outline for how to develop your own metacognitive strategies, and it also provides a list of field-tested metacognitive strategies categorized by math concept area. You can access any of the listed topics simply by clicking on the appropriate title. This site complements the instructional video model for Teaching Metacognitive Strategies, which you can access by clicking on the Instructional Strategies site found on the main navigational page. You can also access the video model by clicking on the icon entitled Metacognitive Strategies Video found in the section titled "How Do I Teach Them."
What Are They?
Math metacognitive strategies are simply memorable plans or approaches that students use to problem-solve. These strategies include the student’s thinking as well as their physical actions (Lenz, Ellis, & Scanlon, 1996). Some of the most common metacognitive strategies come in the form of mnemonics, which are meaningful words where the letters in the word each stand for a step in a problem-solving process or for important pieces of information about a particular topic of interest. For example, you may remember the names of the Great Lakes through the mnemonic "HOMES:" Huron, Ontario, Michigan, Erie, Superior. Metacognitive strategies can also come in the form of easy to remember phrases or through pictures that are easy to recall. Many of us learned the Order of Operations through the strategy phrase, "Please Excuse My Dear Aunt Sally": Parentheses, Exponents, Multiplication/Division, Addition/Subtraction.
Two important characteristics of any effective metacognitive strategy are that it must be memorable and it must accurately represent the learning task. It also can be helpful for students who have learning problems when the form the strategy takes has some meaningful connection to its corresponding learning task. For example, the mnemonic, "DRAW," is a mnemonic that provides students the steps necessary for drawing the solutions to basic computation problems. The word "DRAW" clearly relates to the learning task of drawing tallies and circles to solve these type problems.
[ back to top ]
How Do They Positively Impact Students Who Have Learning Problems?
Metacognitive strategies positively impact students who have learning problems because they provide these students an efficient way to acquire, store, and express information and skills (Mercer & Mercer, 1993). For many students who have learning problems, their inability to efficiently retrieve information previously stored in memory negatively impacts their ability to accurately express what they know. Well developed metacognitive strategies aide such information retrieval for these students. As you have learned in the section entitled "Math Learning Problems," students who have learning problems tend to be passive learners. While the reasons for this learning characteristic may differ based on students’ individual learning problems (e.g. memory problems, cognitive processing difficulties, learned helplessness), effective metacognitive strategies can be of substantial benefit to students who have a variety of learning problems. The key to the success of metacognitive strategies is that when they are taught appropriately, they assist learners who are dependent on high levels of teacher support to become independent learners. When students have been directly taught the strategy, the strategy’s purpose, how to use the strategy, and are provided opportunities to practice using the strategy, these students posses a powerful learning tool that builds learning independence. Confronted with a problem-solving situation, these students can now implement the appropriate metacognitive strategy when they have difficulty remembering how to solve a particular problem. Therefore, instead of relying on the teacher for assistance, they can independently help themselves. This can be a tremendously invigorating feeling for students (and teachers)!
One possible misconception some educators have about metacognitive strategies is that they believe metacognitive strategies are "crutches" that actually make students dependent learners – dependent on the strategy. Metacognitive strategies that are "written out" and taught, are simply formalized versions of "naturally occurring" problem-solving strategies that high achieving students use everyday. While some students seem to use strategies without much instruction or prompting, it must be remembered that this is not true for all students. Depriving these students of problem-solving strategies when they can be easily taught would be an educational travesty. As students learn, practice, and independently use metacognitive strategies, these strategies often become integrated into these students’ learning repertoires.
[ back to top ]
Do I Need to Teach Them?
Yes! A potential misconception about metacognitive strategies is that they, in and of themselves, cure learning problems. This perception may lead some teachers to simply supply the student with a copy of a strategy without directly teaching the strategy to the student. When the student does not use the strategy, or doesn’t use it appropriately, the teacher may conclude that the metacognitive strategy is not helpful. It is important to remember that metacognitive strategies are like any skill we want students to learn. Metacognitive strategies need to be taught! This is especially true for students who have learning problems.
[ back to top ]
How Do I Teach Them?
Strategies should be directly taught to students who have learning problems. The use of explicit teacher modeling is the most effective way to ensure that these students will understand the purpose of a metacognitive strategy, how to use it, and under what circumstances it should be used (Lenz, Ellis, & Scanlon, 1996). Providing students with practice opportunities using the metacognitive strategy is also an important component of metacognitive strategy instruction. It is also very helpful to provide students visual cues of the strategy in the classroom. The strategy can be posted on classroom walls or on a bulletin board; strategies can also be written on individual cue sheets. Students can then keep them at their desk or in their math folder. Some students may also benefit from keeping a notebook that contains all of the math strategies that student has learned. This "strategy notebook" can be organized by math concept/skill and could be used by the student as a resource when they are working independently in class or at home. While such cueing is very helpful for students who have learning problems initially, many students begin to internalize the strategy with continued practice. However, other students may need their strategy notebook for longer periods of time due to significant memory problems.
return
When Should They be Taught?
Metacognitive strategies should be taught after the student has acquired an understanding of the math concept/skill. Strategies are not intended to be substitutes for conceptual understanding!
[ back to top ]
Important Tips for Implementing Metacognitive Strategies
- Strategies should be implemented after the student has acquired an understanding of the concept/skill.
- Strategies must be taught, using explicit systematic instruction.
- Strategies provide students the opportunity to practice independently, which, in turn, builds fluency and mastery of the skill.
- Strategies can be especially helpful for students who are having difficulty moving from the representational level of understanding to the abstract level of understanding because they allow students to independently practice problem solving at the abstract level. Successful practice provides the repetition students often need to establish a working memory of the concept or skill.
- Student use and performance using strategies should be monitored.
- Strategies, in and of themselves, are not magic. It is how you teach them and incorporate their use in your students’ learning that is key!
[ back to top ]
How Can I Develop a Learning Strategy For My Students?
Follow the steps listed below to develop a metacognitive strategy that will be specific for the skill you are teaching.
- Do a task analysis of the math skill the student(s) is learning.
- After breaking the math skill down into its essential subskills/tasks, examine the skills for the "major" subskills that carry out the essential elements of the skill that is the focus of the learning strategy; or, look for ways to chunk the subskills into meaningful parts.
- Give each major subskill, or "chunk" of subskills a general name, title, or phrase that gives the main idea of what the grouped subskills within each "chunk" generally do.
- These chunks or parts are the major steps of your learning strategy. It is essential that the number of steps you incorporate into your learning strategy range from 3 to 6, depending on the complexity and nature of the skill.
- Decide the appropriate order of the steps. For most math skills, there is a definite sequential hierarchy.
- Develop a meaningful way for the student(s) to remember the steps of the strategy. It is helpful that the form the strategy takes is meaningful to the student. Relating strategies to things that are relevant to students’ lives is an effective way to do this.
[ back to top ]
Mnemonics
A memorable word or phrase in which each letter of the word or the first letter of each word in a phrase stands for a step in the strategy.
S it up straight
L ean forward
A sk questions
N od your head
T rack the teacher
- or -
Sally Likes All Nice Teachers
Steps for developing a mnemonic device:
F I R S T (Mercer & Mercer, 1998)
1. F orm a word(that incorporates important parts of skill)-HOMES for name of each Great Lake
2. I nsert extra letters to form a mnemonic word if needed. (e.g. BrACE for remembering scientific objects that have never been seen – black holes, antimatter, cosmic rays, & earth’s core.)
3. R earrange letters to form a mnemonic word (when order is not important)
4. S hape a sentence to form a mnemonic (see above example "Sally Likes All Nice Teachers")
5. T ry combinations of first four steps to create a mnemonic.
Picture Cues
For students with significant reading and/or memory problems, picture cues can be added to each step in order to make the words more meaningful/memorable. With the mnemonic SLANT, for example, a picture of someone sitting up straight in the chair could be used with the "S" step. Sometimes, picture cues can be used for one or two especially problematic steps that students have difficulty remembering or for steps you really want to emphasize.
Visual Displays
Using graphic organizers such as semantic webs, concept charts, flow charts, or pictures of objects with labeled parts can be especially helpful as study strategies.
[ back to top ]
Concept Areas
Click on any of the math concept areas listed below to access descriptions and examples for the appropriate metacognitive strategy(ies). Each of the strategies listed below are research based and/or have been successfully field-tested by teachers.
Basic Computations (+, -, x , /)
DRAW (Mercer & Mercer, 1998) – provides students a strategy for solving addition, subtraction, multiplication, & division problems at the representational (by drawing) level or at the abstract level ("in" student’s "head"). Students who don’t need to draw the solution can bypass the draw process and move directly to writing the answer.
D iscover the sign: student finds, circles, and says name of computation sign.
R ead the problem: student reads equation.
A nswer, or draw tallies and/or circles and check your answer: see draw examples for each operation below.
W rite the answer: student writes answer to problem.
Example: Addition
Example: Subtraction

Example: Multiplication
4 x 5 = ___ – "four groups of five equals…"
1. student represents groups with circles.
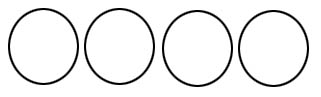
2. student represents objects within groups with tallies.

3. student totals tallies and writes answer.
4 x 5 = 20 – "four groups of five equals twenty"
Example: Division
24 / 4 = ___
1. student draws tallies to represent dividend ("24").
| | | | | | | | | | | | | | | | | | | | | | | |
2. student circles tallies by the value of the divisor ("4").
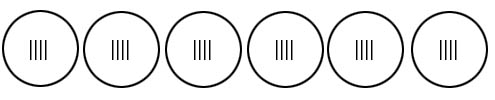
3. student counts number of circles that represent the quotient ("6")
24 / 4 = 6
Count On Big – can be used to solve basic addition problems. Student places finger on the number of greatest value, or "biggest" number, and counts on.
Example:

Count Back Big – can be used to solve basic subtraction problems. Student places finger on the number of greatest value, or "biggest" number, and counts back.
Example:
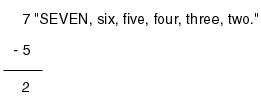
Long Division Face (with Goatee) (Mercer & Mercer, 1998)– students can draw this visual cue which guides them procedurally when solving "long division" problems. * The term "goatee" may be meaningful to your students and this term can remind the to "bring down" after the subtraction step in long division.
Example:
1. Divide
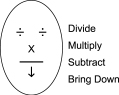
[ back to top ]
Place Value
FIND (Mercer & Mercer, 1998) – helps students determine place value at the abstract level.
F ind the columns (between the numerals).
I nsert the t’s.
N ame the columns (by place value)
D etermine the place value of individual numbers.
Example:

[ back to top ]
Greater Than & Less Than
Gator or Duck Picture Cue (Bernard, 1990).
The gator or duck has to open their mouths wider for the "larger" number.
Example:
- Cut out pictures of gator, duck, or other memorable character whose jaws or mouth open wide
- Paste two together (an ice-cream stick can be inserted between for holding).
- Initially use gator or duck to teach identifying greater than/less than (tell students that the gator or duck has to open their mouth wider for the number that has the greatest value).
- Students use pictures to identify larger number.Teach association of "<" & ">" to pictures.
- Fade pictures to use of symbols only.
- Encourage children to think of pictures when using "<" & ">."
- Provide the pictures as cues for students who need them periodically.
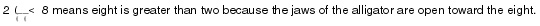
[ back to top ]
Word/Story Problems
FASTDRAW (Mercer & Mercer, 1998)
F ind what you are solving for.
A sk yourself what is the important information
S et up the equation.
T ie down the sign.
D iscover the sign.
R ead the problem.
A nswer, or draw tallies / circles and check.
W rite the answer.
Example:
Sam and Maria went to the music store to buy some of their favorite CD’s. Sam has 3CD's and
and Maria has 4CD's. How many CD’s do they have altogether?
F ind what your are solving for: student underlines the question.
A sk yourself, what is the important information: student finds and circles the number phrases.
S et up the equation: student sets up equation – 3 CD’s __ 4 CD’s =
T ie down the sign: 3 CD’s + 4 CD’s =
*When the equation is set up, then the student uses DRAW to find the solution. See DRAW example above
Other Word/Story Problem Solving Strategies
RIDE (Mercer & Mercer, 1993)
R ead the problem correctly.
I dentify the relevant information
D etermine the operation and unit for expressing the answer.
E nter the correct numbers & calculate, then check the answer.
Problem Solving Steps (Montague & Bos, 1986)
- Read the problem correctly
- Paraphrase the problem orally.
- Visualize, graphically display, or draw a picture of the problem.
- State the problem.
- Hypothesize
- Estimate
- Calculate
- Self-check
SQRQCQ (Strichart, Mangrum, & Iannuzzi, 1998)
S urvey – Read the entire word problem carefully to learn what it is about. If necessary, read it again. Ask your teacher to pronounce or tell you the meaning of any word or term you do not know. Be sure you understand everything in the word problem before you get to the next step.
Q uestion - Ask, "What question needs to be answered in the problem?" Think about what the problem is asking. You may find it helpful to form a picture of the problem in your mind or to draw a picture of the problem. Reading the problem out loud may also help you to identify the question.
R ead - Read to find all the facts you need to answer the question. Look for key words and terms like how much more, how many were left, all together, total, difference that can help you to decide which facts are needed to answer the question. Ignore any information that is not needed to answer the question. Cross out any information not needed.
Q uestion - Ask, "What computations must I so to answer the question?" Decide if you need to add, subtract, multiply, divide, or do these operations in some combination.
C ompute - Set up the problem on paper and do the computations. Check to make sure your computations are accurate. Double-check your work. Circle your answer.
Q uestion - Ask, "Does my answer make sense?" You can tell if it does by going back and looking at the question you tried to answer. Sometimes you will find that your answer could not be correct because it does not fit the facts in the problem. When this happens, go back through the steps of SQCQRQ until you arrive at an answer that does make sense.
SOLVE (Enright Math Systems)
The SOLVE strategy is a general problem solving strategy that can be applied to a variety of word problems including whole numbers, fractions, and decimals.
S tudy the problem.
O rganize the facts.
L ine up the plan.
V erify your plan with computation.
E xamine your answer.
[ back to top ]
Order of Operations
ORDER (Allsopp, 1999) – ORDER is used to assist students who are initially learning to solve multi-operation problems that require understanding of the order of operations. The Rule of Order helps students remember which operation should be solved first. The first letter in each word of the phrase, Many Dogs Are Smelly!, stands for one of the basic math operations (multiplication & division come before addition & subtraction).
Example:
O bserve the problem
R ead the signs.
D ecide which operation to do first.
E xecute the rule of order (Many Dogs Are Smelly!)
R elax, you're done!
A picture cue can be added when students begin working with multi-operation problems that include parentheses, brackets, & braces. Students use this picture cue at the E step of the ORDER Strategy. The picture cue denotes the order for solving operations within parentheses, braces, & brackets. Parentheses (nose of the face) are first, braces (eyes of the face) are second, and brackets (ears of the face) are last.
Example:
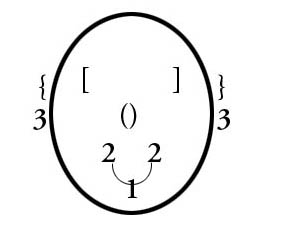
[ back to top ]
Adding Positive and Negative Integers
ADD (Allsopp, 1999) – ADD is used to help students who have established a concrete understanding of the process for adding positive and negative integers and are ready to solve these problems at the abstract level. Each step of the strategy cues students to complete an important component of the problem-solving process.
A sk yourself, is this an addition problem?
D ecide what signs are given
D etermine the problem, what to do, and the sign of the sum.
Example:
| 9 + -6 = _ |
1. A – student looks for addition sign. |
| 9 + -6 = _ |
2. D – student determines the sign for each integer and circles the negative integer. |
| 9 + -6 = 3 |
3. D – student decides 3 things:
1) whether the problem adds a positive and a negative integer or two negative integers;
2) whether to subtract the absolute values of the integers (if they are positive and negative) or add their absolute values ( if both are negative integers);
3) the student determines what sign the sum takes (negative if integers are negative or the sign of the integer that has the greatest absolute value).
|
[ back to top ]
One-Variable Algebraic Equations
DRAW (Mercer, 1994; Allsopp, 1999) – DRAW is used to solve one-variable algebraic equations at the representational and abstract levels of understanding. This mnemonic will be familiar to students who have used DRAW to solve basic addition, subtraction, multiplication, & division problems. However, the steps are different and guide students through the process for solving one-variable algebraic equations.
D iscover the variable, the operations, and what the left side of the equation equals.
R ead the equation and combine like terms on each side of the equation.
A nswer the equation, or draw and check.
W rite the answer for the variable and check the equation.
Example:
How to draw the solution:
4x + 2x = 12
1. Represent the variable "x" with circles. By combining like terms, there are six "x’s."
6x = 12
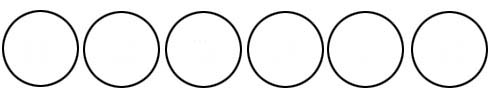
2. The total, "12," is divided equally among the circles.
x = 12/6
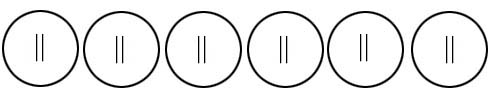
3. The solution is the number of tallies represented in one circle – the variable ‘x."
x = 2

[ back to top ]
|



